October 26, 2015
Modeling Domes
Originally published October 25, 2004
Image Credit: Raffaello Lena and Cristian Fattinnanzi, Fabio Lottero and KC Pau |
|
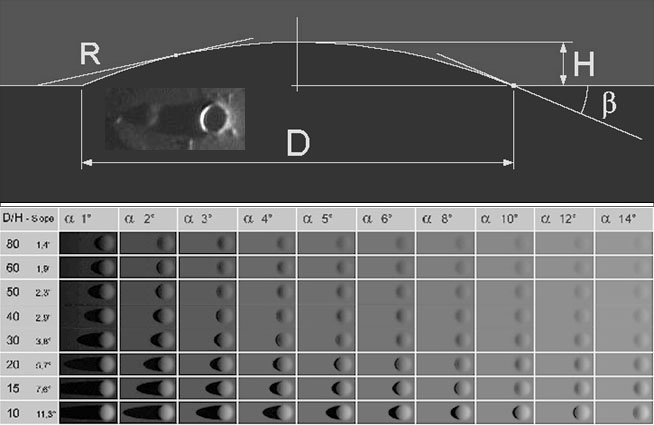
Modeling Domes Domes are one of the most difficult types of lunar objects to study quantitatively. Craters can have their diameters and depths measured fairly directly, but domes are such low and gently sloped features that it is very difficult to measure accurately their shadow lengths to derive topographic information. Members of the Geological Lunar Research Group have developed another way to approach this problem. They have mathematically investigated the relationship between how an ideal hemispherical dome's shadow length (R) varies with solar elevation and with its diameter (D) and height (H) ratio. The bottom part of the illustration shows the computed shadow lengths for various dome D/H ratios (and resulting slopes) against increasing solar elevation (alpha). The graph passes the common sense test - it shows that steep domes (D/H = 10) cast longer shadows and cast them at higher sun angles than low angle domes (D/H = 80). With this table you can estimate (or measure) which D/H value most matches a real dome. For the example of Milichius Pi (in the small excerpt from a KC Pau image taken when the sun was 1.2 degrees above Pi), the best fit is for D/H = 40, yielding a dome slope of 2.9 degrees and a height of about 200 m. Related Links: Yesterday's LPOD: A Long, Cold Mare Tomorrow's LPOD: Eclipse Preview |
|
Author & Editor: COMMENTS?Register, Log in, and join in the comments.
|